Difference between revisions of "M(3,1,2)"

From Block library
Jump to: navigation, search
(Created page with "{{blockbox |title = {{subst:PAGENAME}} |image = |representative = <math>kS_3</math> |defect = <math>kC_3</math> |inertialquotients = <math>C_2</math> |k(B) = 3 |l(B) = 2 |k-...")
 
 
(One intermediate revision by the same user not shown)
Line 1: Line 1:
 +
 
{{blockbox
 
{{blockbox
−
|title = M(3,1,2)
+
|title = M(3,1,2) - <math>kS_3</math>
−
|image =  
+
|image = M(3,1,2)quiver.png
 
|representative = <math>kS_3</math>  
 
|representative = <math>kS_3</math>  
−
|defect = <math>kC_3</math>
+
|defect = [[C3|<math>C_3</math>]]
 
|inertialquotients = <math>C_2</math>
 
|inertialquotients = <math>C_2</math>
 
|k(B) = 3
 
|k(B) = 3
 
|l(B) = 2
 
|l(B) = 2
 
|k-morita-frob = 1
 
|k-morita-frob = 1
 +
|O-morita-frob = 1
 
|cartan = <math>\left( \begin{array}{cc}
 
|cartan = <math>\left( \begin{array}{cc}
 
2 & 1 \\
 
2 & 1 \\
 
1 & 2 \\
 
1 & 2 \\
 
\end{array} \right)</math>
 
\end{array} \right)</math>
 +
|O-morita? = Yes
 +
|O-morita = <math>\mathcal{O} S_3</math>
 +
|source? = Yes
 +
|sourcereps= <math>kS_3</math>
 +
|defect-morita-inv? = Yes
 +
|inertial-morita-inv? = Yes
 +
|decomp = <math>\left( \begin{array}{cc}
 +
1 & 0 \\
 +
0 & 1 \\
 +
1 & 1 \\
 +
\end{array}\right)</math>
 +
|Pic-O = <math>\mathcal{T}(B)=C_2</math>
 +
|k-derived-known? = Yes
 +
|k-derived = [[M(3,1,1)]]
 +
|O-derived-known? = Yes
 +
|Pic-k =
 +
|coveringblocks = [[M(3,1,1)]]
 +
|coveredblocks = [[M(3,1,1)]]
 
}}
 
}}
 +
 +
These are very frequently occuring blocks with [[Blocks with cyclic defect groups|cyclic defect groups]], so are described in work culminating in [[References|[Li96] ]].
 +
 +
== Basic algebra ==
 +
 +
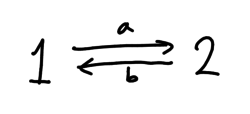
'''Quiver:''' a: <1,2>, b: <2,1>
 +
 +
'''Relations w.r.t. <math>k</math>:''' aba=bab=0
 +
 +
== Other notatable representatives ==
 +
 +
== Covering blocks and covered blocks ==
 +
 +
Let <math>N \triangleleft G</math> with <math>p'</math>-index and let <math>B</math> be a block of <math>\mathcal{O} G</math> covering a block <math>b</math> of <math>\mathcal{O} N</math>.
 +
 +
If <math>b</math> lies in M(3,1,2), then <math>B</math> must lie in M(3,1,1) or M(3,1,2). <span style="color: red">Example needed.</span>
 +
 +
If <math>B</math> lies in M(3,1,2), then <math>b</math> must lie in M(3,1,1) or M(3,1,2). For example consider the principal blocks of <math>C_3 \triangleleft S_3</math>.
 +
 +
== Projective indecomposable modules ==
 +
 +
Labelling the simple <math>B</math>-modules by <math>S_1, S_2</math>, the projective indecomposable modules have Loewy structure as follows:
 +
 +
<math>\begin{array}{cc}
 +
  \begin{array}{c}
 +
      S_1 \\
 +
      S_2 \\
 +
      S_1 \\
 +
  \end{array}, &
 +
\begin{array}{c}
 +
      S_2 \\
 +
      S_1 \\
 +
      S_2 \\
 +
  \end{array}
 +
\end{array}
 +
</math>
 +
 +
== Irreducible characters ==
 +
 +
All irreducible characters have height zero.

Latest revision as of 21:30, 9 September 2018

M(3,1,2) - [math]kS_3[/math]
M(3,1,2)quiver.png
Representative: [math]kS_3[/math]
Defect groups: [math]C_3[/math]
Inertial quotients: [math]C_2[/math]
[math]k(B)=[/math] 3
[math]l(B)=[/math] 2
[math]{\rm mf}_k(B)=[/math] 1
[math]{\rm Pic}_k(B)=[/math]
Cartan matrix: [math]\left( \begin{array}{cc} 2 & 1 \\ 1 & 2 \\ \end{array} \right)[/math]
Defect group Morita invariant? Yes
Inertial quotient Morita invariant? Yes
[math]\mathcal{O}[/math]-Morita classes known? Yes
[math]\mathcal{O}[/math]-Morita classes: [math]\mathcal{O} S_3[/math]
Decomposition matrices: [math]\left( \begin{array}{cc} 1 & 0 \\ 0 & 1 \\ 1 & 1 \\ \end{array}\right)[/math]
[math]{\rm mf}_\mathcal{O}(B)=[/math] 1
[math]{\rm Pic}_{\mathcal{O}}(B)=[/math] [math]\mathcal{T}(B)=C_2[/math]
[math]PI(B)=[/math] {{{PIgroup}}}
Source algebras known? Yes
Source algebra reps: [math]kS_3[/math]
[math]k[/math]-derived equiv. classes known? Yes
[math]k[/math]-derived equivalent to: M(3,1,1)
[math]\mathcal{O}[/math]-derived equiv. classes known? Yes
[math]p'[/math]-index covering blocks: M(3,1,1)
[math]p'[/math]-index covered blocks: M(3,1,1)
Index [math]p[/math] covering blocks: {{{pcoveringblocks}}}

These are very frequently occuring blocks with cyclic defect groups, so are described in work culminating in [Li96] .

Basic algebra

Quiver: a: <1,2>, b: <2,1>

Relations w.r.t. [math]k[/math]: aba=bab=0

Other notatable representatives

Covering blocks and covered blocks

Let [math]N \triangleleft G[/math] with [math]p'[/math]-index and let [math]B[/math] be a block of [math]\mathcal{O} G[/math] covering a block [math]b[/math] of [math]\mathcal{O} N[/math].

If [math]b[/math] lies in M(3,1,2), then [math]B[/math] must lie in M(3,1,1) or M(3,1,2). Example needed.

If [math]B[/math] lies in M(3,1,2), then [math]b[/math] must lie in M(3,1,1) or M(3,1,2). For example consider the principal blocks of [math]C_3 \triangleleft S_3[/math].

Projective indecomposable modules

Labelling the simple [math]B[/math]-modules by [math]S_1, S_2[/math], the projective indecomposable modules have Loewy structure as follows:

[math]\begin{array}{cc} \begin{array}{c} S_1 \\ S_2 \\ S_1 \\ \end{array}, & \begin{array}{c} S_2 \\ S_1 \\ S_2 \\ \end{array} \end{array} [/math]

Irreducible characters

All irreducible characters have height zero.

Retrieved from ‘http://wiki.manchester.ac.uk/blocks/index.php?title=M(3,1,2)&oldid=372’