M(8,5,5)

M(8,5,5) - [math]B_0(kSL_2(8))[/math]

Representative:	[math]B_0(kSL_2(8))[/math]
Defect groups:	[math]C_2 \times C_2 \times C_2[/math]
Inertial quotients:	[math]C_7[/math]
[math]k(B)=[/math]	8
[math]l(B)=[/math]	7
[math]{\rm mf}_k(B)=[/math]	1
[math]{\rm Pic}_k(B)=[/math]
Cartan matrix:	[math]\left( \begin{array}{ccccccc} 8 & 4 & 4 & 4 & 2 & 2 & 2 \\ 4 & 4 & 2 & 2 & 0 & 2 & 1 \\ 4 & 2 & 4 & 2 & 1 & 0 & 2 \\ 4 & 2 & 2 & 4 & 2 & 1 & 0 \\ 2 & 0 & 1 & 2 & 2 & 0 & 0 \\ 2 & 2 & 0 & 1 & 0 & 2 & 0 \\ 2 & 1 & 2 & 0 & 0 & 0 & 2 \\ \end{array} \right)[/math]
Defect group Morita invariant?	Yes
Inertial quotient Morita invariant?	Yes
[math]\mathcal{O}[/math]-Morita classes known?	Yes
[math]\mathcal{O}[/math]-Morita classes:	[math]B_0(\mathcal{O}SL_2(8))[/math]
Decomposition matrices:	[math]\left( \begin{array}{ccccccc} 1 & 0 & 0 & 0 & 0 & 0 & 0 \\ 1 & 1 & 1 & 1 & 0 & 0 & 0 \\ 1 & 0 & 1 & 0 & 0 & 0 & 1 \\ 1 & 0 & 0 & 1 & 1 & 0 & 0 \\ 1 & 1 & 0 & 0 & 0 & 1 & 0 \\ 1 & 1 & 1 & 0 & 0 & 0 & 1 \\ 1 & 0 & 1 & 1 & 1 & 0 & 0 \\ 1 & 1 & 0 & 1 & 0 & 1 & 0 \\ \end{array}\right)[/math]
[math]{\rm mf}_\mathcal{O}(B)=[/math]	1
[math]{\rm Pic}_{\mathcal{O}}(B)=[/math]	[math]C_3[/math]
[math]PI(B)=[/math]	{{{PIgroup}}}
Source algebras known?	No
Source algebra reps:
[math]k[/math]-derived equiv. classes known?	Yes
[math]k[/math]-derived equivalent to:	M(8,5,4)
[math]\mathcal{O}[/math]-derived equiv. classes known?	Yes
[math]p'[/math]-index covering blocks:	M(8,5,8)
[math]p'[/math]-index covered blocks:	Potentially M(8,5,8)
Index [math]p[/math] covering blocks:	{{{pcoveringblocks}}}

The projective indecomposable modules of the [math]2[/math]-blocks of the groups [math]SL_2(2^n)[/math] were computed by Alperin in [Al79] and the quiver and relations by Koshita in [Ko94]. A splendid derived equivalence with M(8,5,4) was constructed by Rouquier in [Ro95].

Basic algebra

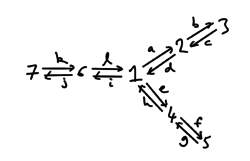
Quiver: a<1,2>, b:<2,3>, c:<3,2>, d:<2,1>, e:<1,4>, f:<4,5>, g:<5,4>, h:<4,1>, i:<1,6>, j:<6,7>, k:<7,6>, l:<6,1>

Relations w.r.t. [math]k[/math]: [math]da=he=li=0[/math], [math]cb=gf=kj=0[/math], [math]bcd=dil[/math], [math]fgh=had[/math], [math]jkl=leh[/math], [math]abc=ila[/math], [math]efg=ade[/math], [math]ijk=ehi[/math], [math]cdi=gha=kle[/math], [math]lab=def=hij[/math]

Irreducible characters

All irreducible characters have height zero.

Notes

Back to [math]C_2 \times C_2 \times C_2[/math]